參數(shù)資料
型號(hào)： AM-3A-000515
廠商： MITEQ INC
元件分類： 放大器
英文描述： 5 MHz - 1500 MHz RF/MICROWAVE WIDE BAND LOW POWER AMPLIFIER
文件頁(yè)數(shù)： 23/25頁(yè)
文件大小： 1906K
代理商： AM-3A-000515
BIPOLAR AMPLIFIERS
OVERALL GAIN WINDOW
An overall gain window specification defines the
absolute minimum and maximum gain values over
both temperature and frequency.
It is the most complete way to specify an amplifier;
however, it also impacts the price due to the addition-
al testing and alignment required from adding this
constraining parameter.
INTERCEPT POINT
Solid state amplifiers use transistors, either bipolar or
field effect, to provide gain. Although these transistors
are generally used in a linear mode (except in the
case of other than a class A amplifier), they still exhib-
it nonlinear phenomenon, such as intermodulation
effects and harmonic generation. These effects are
evident in spurious products present at the output. In
the case of the single-tone condition, the spurious sig-
nals are the harmonics of the fundamental input sig-
nal. In the case of the two-tone condition, the spuri-
ous signals are a mixing product of two input signals
at the frequencies f1 and the other at f2. The most
commonly discussed being the second order and the
third order two-tone spurs.
Second order two-tone spurs are the sum and differ-
ence product of the fundamental input frequencies,
i.e.,
fSPUR = f1 ±f2
These spurious signals are only of concern when the
band is greater than one octave.
If the frequency
range is less than one octave, the two-tone second
order spurs will be out of band.
SPECIFICATION DEFINITIONS (CONT.)
These spurious signals are characterized with respect
to the input signal by means of a theoretical tool
called an intercept point. These points are defined as
the point where the linear curve of input vs. output
power of the fundamental would intersect with the lin-
ear curve of the spurious signal if saturation effects
would not limit the output levels of these signals.
Since it is known that the second order spurious
products have a slope of 2:1 with respect to the fun-
damental input power, the value of the spurs can be
estimated if the input signal power (PIN) and the out-
put second order intercept point (OIP2) are known.
The relationship is as follows:
Two-Tone Second Order
Spurious Suppression = OIP2 - (PIN+ G)
Two-Tone Second Order
Spurious Level = 2 (PIN+ G) - OIP2
Third order spurious products result from combinations
of the fundamental signal and the second harmonics.
fSPUR = |2f1 ±f2| ± |f1 ±2f2|
The slope of the third order spurious signals is 3:1
with respect to the fundamental input power, and
again the value of the spurs can be estimated if the
input signal power (PIN) and the output third order
intercept point (OIP3) are known. The relationship is
as follows:
Two-Tone Third Order = 2 {OIP3 - (PIN+ G)}
Spurious Suppression
Two-Tone Third Order = 3 (PIN+ G) - 2 OIP3
Spurious Level
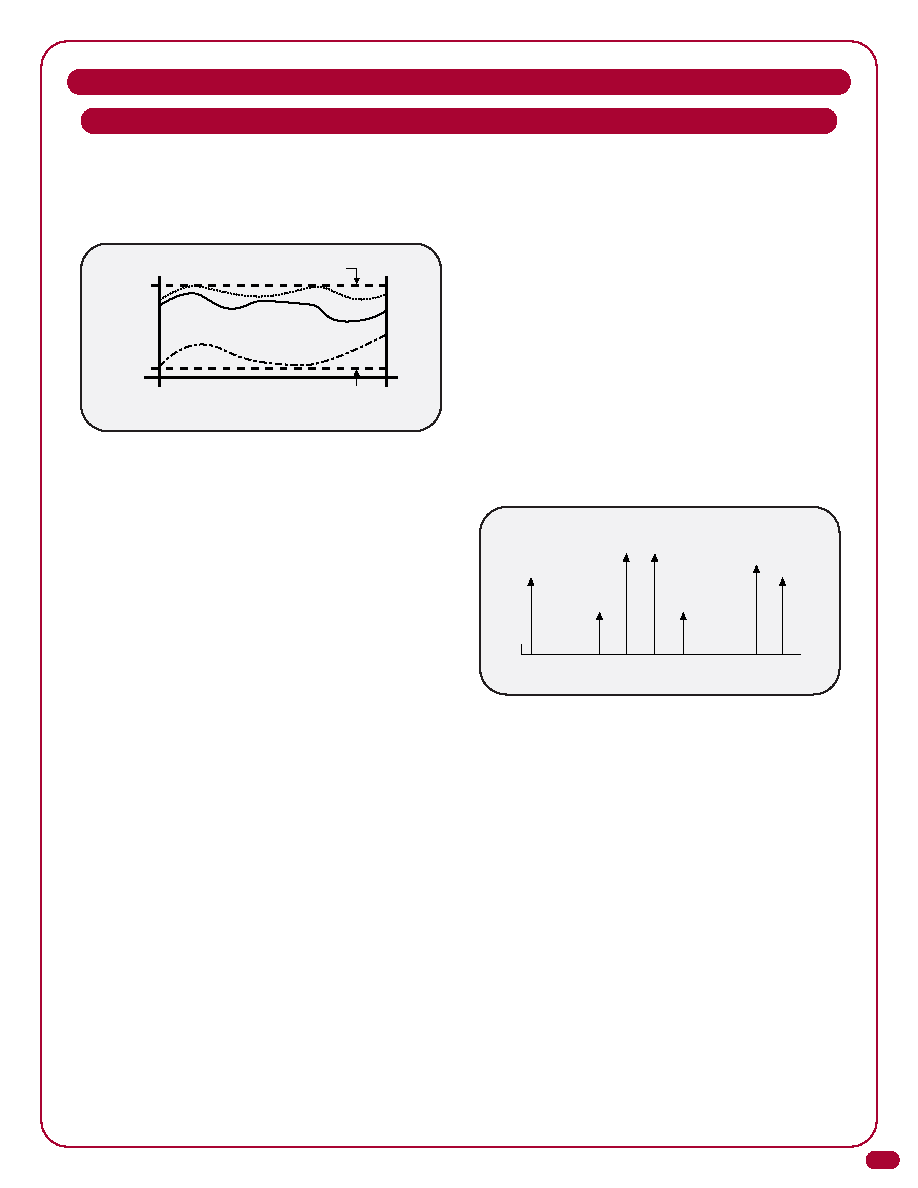
Peak-to-Peak Gain Flatness
Operating Frequency Range
(Measured at room, hot and cold)
Max. Gain
Min. Gain
F Low
F High
Two
Input
Tones
f2-f1
f1-f2
2f1-f2
2f2-f1
f1
2f1
f2
2nd Order
3rd Order
2nd Harm.
Intermodulation Spurious Frequency Spectrum
6
相關(guān)PDF資料
PDF描述
AU-1241-70 50 MHz - 90 MHz RF/MICROWAVE NARROW BAND LOW POWER AMPLIFIER
AUP-1479 5 MHz - 300 MHz RF/MICROWAVE WIDE BAND MEDIUM POWER AMPLIFIER
AM-1571-140 100 MHz - 180 MHz RF/MICROWAVE NARROW BAND LOW POWER AMPLIFIER
AM-1571-70 50 MHz - 90 MHz RF/MICROWAVE NARROW BAND LOW POWER AMPLIFIER
AU-2A-1241 50 MHz - 90 MHz RF/MICROWAVE NARROW BAND LOW POWER AMPLIFIER
相關(guān)代理商/技術(shù)參數(shù)
參數(shù)描述
AM3A026C 制造商:MAG-LITE 功能描述:
AM3A064 制造商:MAG-LITE 功能描述:
AM3A26A8A8A30-250VAC-20 制造商:Heinemann / Eaton 功能描述:
AM3-A2A2A3-A-30-3 制造商:Heinemann / Eaton 功能描述:
AM3-A39-A-10-252 制造商:Heinemann / Eaton 功能描述:
發(fā)布緊急采購(gòu)，3分鐘左右您將得到回復(fù)。

采購(gòu)需求

(若只采購(gòu)一條型號(hào)，填寫(xiě)一行即可)

發(fā)布成功！您可以繼續(xù)發(fā)布采購(gòu)。也可以進(jìn)入我的后臺(tái)，查看報(bào)價(jià)

發(fā)布成功！您可以繼續(xù)發(fā)布采購(gòu)。也可以進(jìn)入我的后臺(tái)，查看報(bào)價(jià)

*型號(hào) *數(shù)量 廠商 批號(hào) 封裝
添加更多采購(gòu)

我的聯(lián)系方式

*
*
*