- 您現(xiàn)在的位置:買賣IC網(wǎng) > PDF目錄358855 > LM3940IS-3.3 (NATIONAL SEMICONDUCTOR CORP) Cap-Free, NMOS, 150mA Low Dropout Regulator with Reverse Current Protection PDF資料下載
參數(shù)資料
| 型號(hào): | LM3940IS-3.3 |
| 廠商: | NATIONAL SEMICONDUCTOR CORP |
| 元件分類: | 基準(zhǔn)電壓源/電流源 |
| 英文描述: | Cap-Free, NMOS, 150mA Low Dropout Regulator with Reverse Current Protection |
| 中文描述: | 3.3 V FIXED POSITIVE LDO REGULATOR, 1 V DROPOUT, PSSO3 |
| 封裝: | TO-263, 3 PIN |
| 文件頁數(shù): | 5/11頁 |
| 文件大小: | 291K |
| 代理商: | LM3940IS-3.3 |
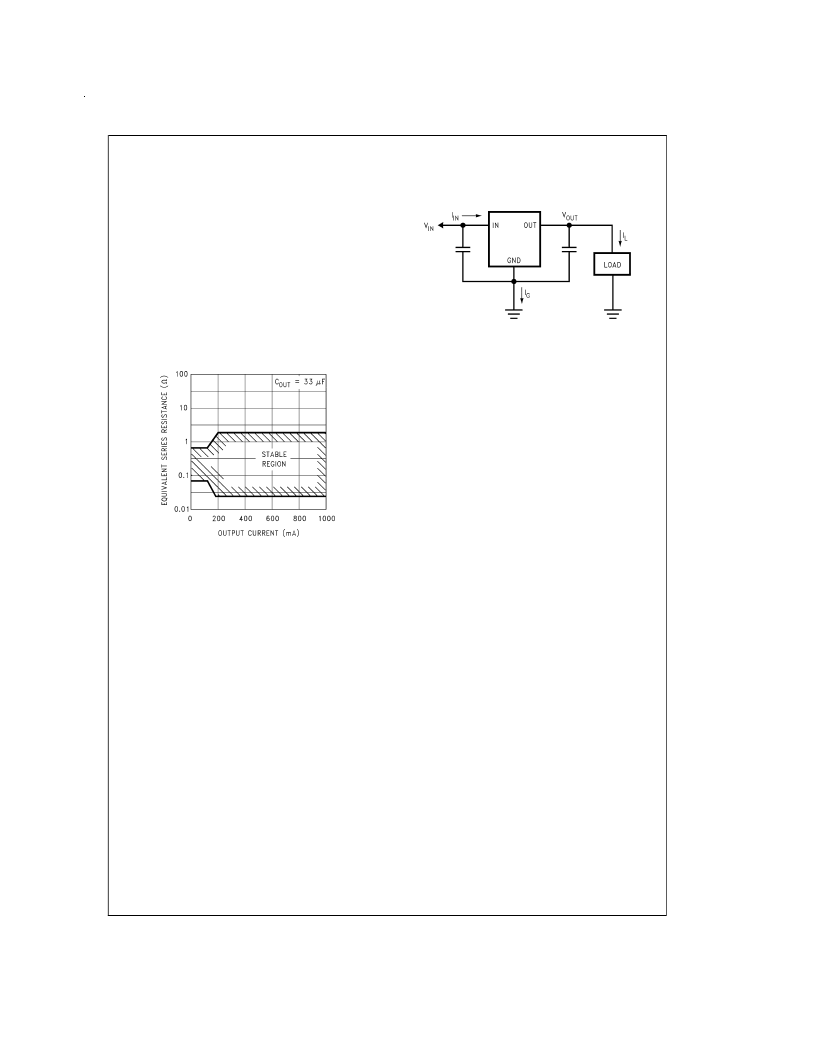
Application Hints
EXTERNAL CAPACITORS
The output capacitor is critical to maintaining regulator stabil-
ity, and must meet the required conditions for both ESR
(Equivalent Series Resistance) and minimum amount of ca-
pacitance.
MINIMUM CAPACITANCE:
The minimum output capacitance required to maintain stabil-
ity is 33 μF (this value may be increased without limit).
Larger values of output capacitance will give improved tran-
sient response.
ESR LIMITS:
The ESR of the output capacitor will cause loop instability if
it is too high or too low. The acceptable range of ESR plotted
versus load current is shown in the graph below.
It is essen-
tial that the output capacitor meet these requirements,
or oscillations can result.
It is important to note that for most capacitors, ESR is speci-
fied only at room temperature. However, the designer must
ensure that the ESR will stay inside the limits shown over the
entire operating temperature range for the design.
For aluminum electrolytic capacitors, ESR will increase by
about 30X as the temperature is reduced from 25C to
40C. This type of capacitor is not well-suited for low tem-
perature operation.
Solid tantalum capacitors have a more stable ESR over tem-
perature, but are more expensive than aluminum electrolyt-
ics. A cost-effective approach sometimes used is to parallel
an aluminum electrolytic with a solid Tantalum, with the total
capacitance split about 75/25% with the Aluminum being the
larger value.
If two capacitors are paralleled, the effective ESR is the par-
allel of the two individual values. The “flatter” ESR of the Tan-
talum will keep the effective ESR from rising as quickly at low
temperatures.
HEATSINKING
A heatsink may be required depending on the maximum
power dissipation and maximum ambient temperature of the
application. Under all possible operating conditions, the junc-
tion temperature must be within the range specified under
Absolute Maximum Ratings.
To determine if a heatsink is required, the power dissipated
by the regulator, P
D
, must be calculated.
The figure below shows the voltages and currents which are
present in the circuit, as well as the formula for calculating
the power dissipated in the regulator:
The next parameter which must be calculated is the maxi-
mum allowable temperature rise, T
R
(max). This is calcu-
lated by using the formula:
T
R
(max) = T
J
(max) T
A
(max)
Where: T
J
(max)
is the maximum allowable junction tem-
perature, which is 125C for commercial
grade parts.
T
A
(max)
is the maximum ambient temperature
which will be encountered in the applica-
tion.
Using the calculated values for T
(max) and P
, the maxi-
mum allowable value for the junction-to-ambient thermal re-
sistance,
θ
(JA)
, can now be found:
θ
(JA)
= T
R
(max)/P
D
IMPORTANT:
If the maximum allowable value for
θ
is
found to be
≥
60C/W for the TO-220 package,
≥
80C/W for
the TO-263 package, or
≥
174C/W for the SOT-223 pack-
age, no heatsink is needed since the package alone will dis-
sipate enough heat to satisfy these requirements.
If the calculated value for
θ
(JA)
falls below these limits, a
heatsink is required.
HEATSINKING TO-220 PACKAGE PARTS
The TO-220 can be attached to a typical heatsink, or se-
cured to a copper plane on a PC board. If a copper plane is
to be used, the values of
θ
will be the same as shown in
the next section for the TO-263.
DS012080-5
FIGURE 1. ESR Limits
DS012080-6
I
IN
= I
L
+ I
G
P
D
= (V
IN
V
OUT
) I
L
+ (V
IN
) I
G
FIGURE 2. Power Dissipation Diagram
www.national.com
5
相關(guān)PDF資料 |
PDF描述 |
|---|---|
| LM3940IMPX-3.3 | THREE-TERMINAL POSITIVE FIXED VOLTAGE REGULATORS |
| LM3940ISX-3.3 | THREE-TERMINAL POSITIVE FIXED VOLTAGE REGULATORS |
| LM3940J-3.3-QML | Cap-Free, NMOS, 150mA Low Dropout Regulator with Reverse Current Protection |
| LM394BH | LM194/LM394 Supermatch Pair |
| LM394CH | LM194/LM394 Supermatch Pair |
相關(guān)代理商/技術(shù)參數(shù) |
參數(shù)描述 |
|---|---|
| LM3940IS33NOPB | 制造商:Texas Instruments 功能描述: |
| LM3940ISX-3.3 | 功能描述:低壓差穩(wěn)壓器 - LDO RoHS:否 制造商:Texas Instruments 最大輸入電壓:36 V 輸出電壓:1.4 V to 20.5 V 回動(dòng)電壓(最大值):307 mV 輸出電流:1 A 負(fù)載調(diào)節(jié):0.3 % 輸出端數(shù)量: 輸出類型:Fixed 最大工作溫度:+ 125 C 安裝風(fēng)格:SMD/SMT 封裝 / 箱體:VQFN-20 |
| LM3940ISX-3.3/NOPB | 功能描述:低壓差穩(wěn)壓器 - LDO 1A LDO REG RoHS:否 制造商:Texas Instruments 最大輸入電壓:36 V 輸出電壓:1.4 V to 20.5 V 回動(dòng)電壓(最大值):307 mV 輸出電流:1 A 負(fù)載調(diào)節(jié):0.3 % 輸出端數(shù)量: 輸出類型:Fixed 最大工作溫度:+ 125 C 安裝風(fēng)格:SMD/SMT 封裝 / 箱體:VQFN-20 |
| LM3940ISX33 | 制造商:Texas Instruments 功能描述: |
| LM3940IT-3.3 | 功能描述:低壓差穩(wěn)壓器 - LDO RoHS:否 制造商:Texas Instruments 最大輸入電壓:36 V 輸出電壓:1.4 V to 20.5 V 回動(dòng)電壓(最大值):307 mV 輸出電流:1 A 負(fù)載調(diào)節(jié):0.3 % 輸出端數(shù)量: 輸出類型:Fixed 最大工作溫度:+ 125 C 安裝風(fēng)格:SMD/SMT 封裝 / 箱體:VQFN-20 |
發(fā)布緊急采購,3分鐘左右您將得到回復(fù)。